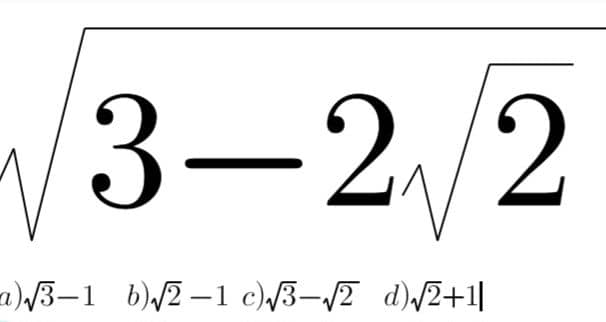
Simplifying the Expression: √3 – 2√2
Let’s break down the expression ( \sqrt{3} – 2\sqrt{2} ) step by step.
Step 1: Rewrite 3 as ( 2 + 1 )
We start by expressing 3 as the sum of 2 and 1
Step 2: Substitute and Simplify
Now, the original expression becomes:
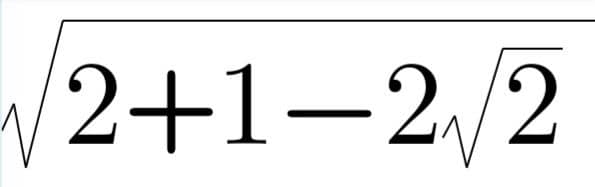
Step 3: Recognize the Perfect Square
Notice that the expression inside the square root can be rewritten as a perfect square:
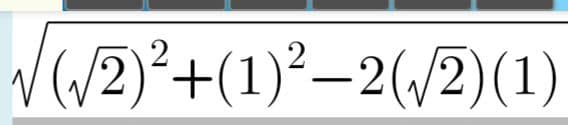
This matches the identity ( a^2 + b^2 – 2ab = (a – b)^2 ), where ( a = \sqrt{2} ) and ( b = 1 ).
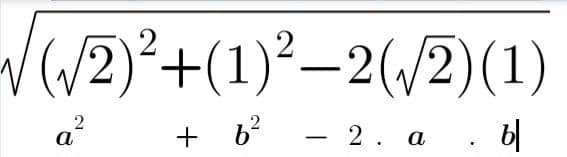
Step 4: Factorize the Expression
Therefore, the expression simplifies to:

Step 5: Simplify Further
Since the square root and the square cancel each other, we obtain:
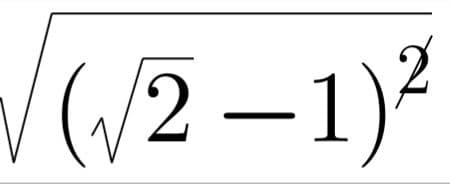
Thus, the simplified form is
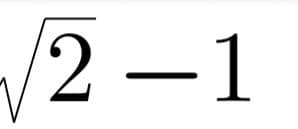
Leave a Reply