A man observes the angle of the elevation of the top of the building to be 30degree. He walks towards it in a horizontal line through its base. On covering 60m, the angle of the elevation changes to 60degrees. Find the height of the building. (heights and distances Example1)

Heights and distances

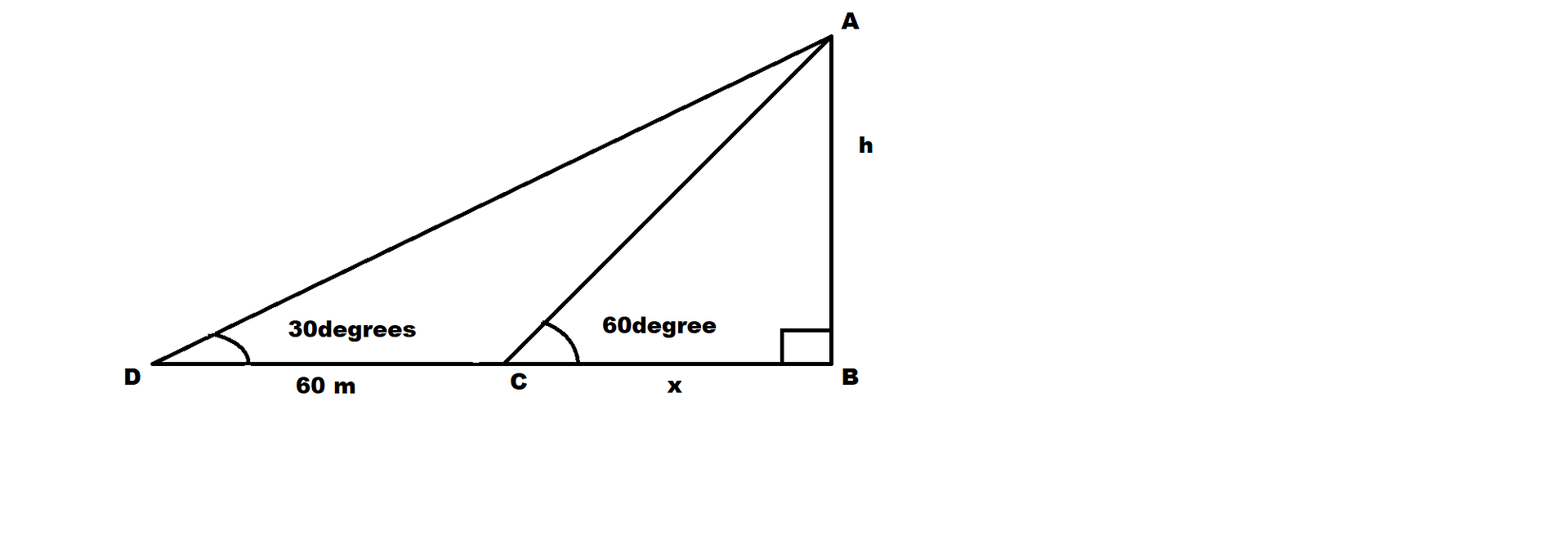
Step 1: Understanding the Geometry:

   Let’s start by assigning labels to the different parts of the problem.  

   – Let AB be the height of the building (denoted as h).  

   D be the initial observation point where the angle of elevation is 30°.  

   C be the second observation point, where the angle of elevation is 60°.  

   – The horizontal distance between points C and D is given as 60 meters.  

   – Let the horizontal distance from the building’s base B to the point D be ‘x’ meters.

 With these designations, we can proceed to apply trigonometry to both triangles formed by the angles of elevation.

Step 2: Using Trigonometry for Triangle ABD (angle 30°):  

   In right-angled triangle ABD, In this case, the tangent of the angle can be written as

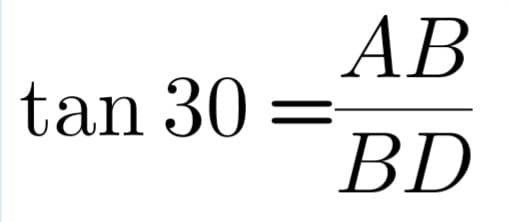
   Substituting (AB = h) and (BD = 60+ x), Thus:

   Solving for h, Accordingly:

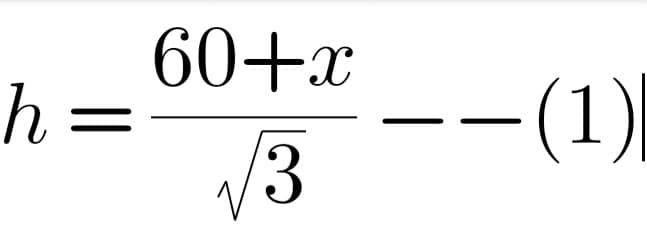
Thus, this equation (1) gives us a relation between the height (h) and the horizontal distance (x).

Step 3: Using Trigonometry for Triangle ABC (angle 60°):  

   Specifically, let’s focus on triangle ABC. In this case, the tangent of 60° is given by:

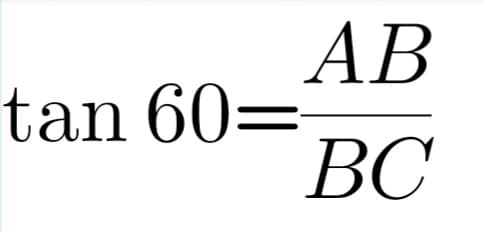
   Substituting AB = h and BC = x , we get:

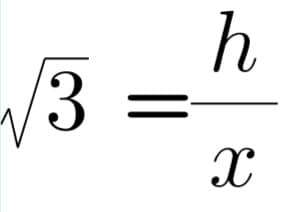
   Solving for h, Thus,

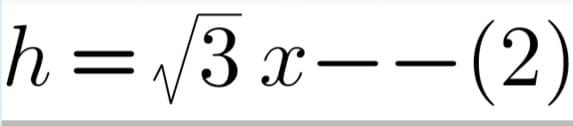
Step 4: Then, Equating the Two Expressions for h:

   Now that we have two expressions for h from equations (1) and (2), we can set them equal to each other:

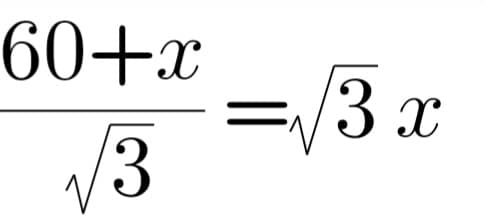
  To simplify, multiply both sides by squareroot(3):

    60 + x = 3x

   Solving for x, we get:

   3x – x = 60

   2x = 60 Thus,

   x = 30

   That is to say, the distance from point D to the base of the building is 30 meters.

Step 5: Hence Find the Height of the Building:

   Finally, substitute x = 30 meters into equation (2) to find the height h:

   

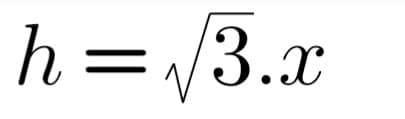
  Using sqrt{3} ~1.732,

As a result:

   h = 30 X 1.732 = 51.96m

Conclusion:

Therefore, the height of the building is approximately 52 m.

Use of trigonometry

Additionally, Arithmetic progression problems