Consider the infinite nested radicals expression:

Let n represent this infinite nested radicals. so the equation becomes:

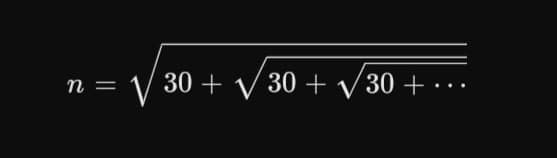
Square both sides to eliminate the square root:

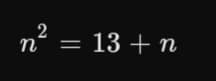
So, Rearrange the equation to form a standard quadratic equation:

n²– n – 30 = 0

We solve this quadratic equation using the quadratic formula:

Since ( sqrt{121} = 11 ),

So,the equation becomes:

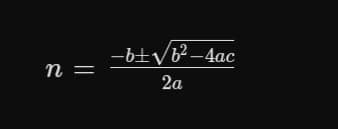
Now,Substituting the values ( a = 1 ), ( b = -1 ), and ( c = -30 ) into the formula

As a result,we get:

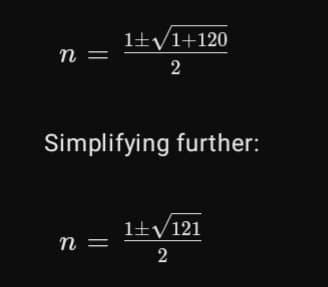
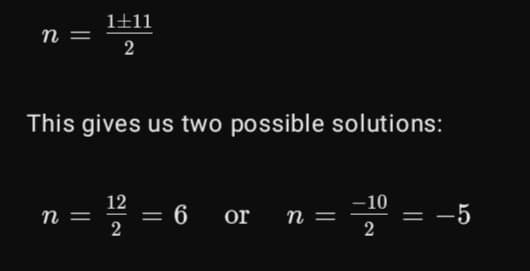
Method 2 :

Also,we can solve the quadratic equation n² – n – 30 = 0 by factorization.

Step 1: Write down the equation

n² – n – 30 = 0

Step 2: Identify coefficients

The equation is of the form ( n² + bn + c = 0 ), where:( a = 1 ) (coefficient of ( n² ))( b = -1 ) (coefficient of ( n ))( c = -30 ) (constant term)

Step 3: Now, Find two numbers that multiply to ( ac ) and add to ( b )

We need to find two numbers that multiply to ( a x c = 1 x -30 = -30 ) as well as add up to (b = -1 ).

The two numbers that satisfy these conditions are ( -6 ) and ( 5 ), because:( -6 x 5 = -30 )( -6 + 5 = -1 )

Step 4: Hence rewrite the middle term using these two numbers

We can now rewrite the quadratic equation by splitting the middle term ( -n ) into ( -6n + 5n ):

n² – 6n + 5n – 30 = 0

Step 5: Hence Factor by groupingGroup the terms into pairs:

(n² – 6n) + (5n – 30) = 0

Factor out the common factors in each group:

n(n – 6) + 5(n – 6) = 0

Step 6: Factor out the common binomial

Since both groups contain the common factor ( (n – 6) ), factor this out:

(n – 6)(n + 5) = 0

Step 7: Solve for ( n )

Set each factor equal to zero and solve for ( n ):

n – 6 = 0 ⟹ n = 6

n + 5 = 0 ⟹ n = -5

However, because ( n ) represents a length (and must be positive), we discard the negative solution.

Thus, the value of ( n ) is:

n = 6

Therefore, for the infinite nested radical expression with the base value 30,

Thus, the solution is 6

Read More